| Derivation of the Timewave from the King Wen Sequence of Hexagrams |
| by Terence McKenna |
Figure 1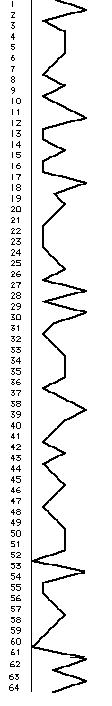 |
The idea that time is experienced as a series of identifiable elements in flux
is highly developed in the I Ching. Indeed the temporal modeling of the I Ching
offers an extremely well-developed alternative to the "flat-duration" point of
view. The I Ching views time as a finite number of distinct and irreducible
elements, in the same way that the chemical elements compose the world of
matter. For the Taoist sages of pre-Han China time was composed of sixty-four
irreducible elements. It is upon relations among these sixty-four elements that
I have sought to erect a new model of time that incorporates the idea of the
conservation of novelty and still recognizes time as a process of becoming.
The earliest arrangement of the hexagrams of the I Ching is the King Wen Sequence. It was this sequence that I chose to study as a possible basis for a new model of the relationship of time to the ingression and conservation of novelty. In studying the kinds of order in the King Wen Sequence of the I Ching I made a number of remarkable discoveries. It is well known that hexagrams in the King Wen sequence occur in pairs. The second member of each pair is obtained by inverting the first. In any sequence of the sixty-four hexagrams there are eight hexagrams which remain unchanged when inverted. In the King Wen Sequence these eight hexagrams are paired with hexagrams in which each line of the first hexagram has become its opposite, (yang changed to yin and vice-versa). The question remains as to what rule or principle governs the arrangement of the thirty-two pairs of hexagrams comprising the King Wen Sequence. My intuition was to look at the first order of difference, that is, how many lines change as one moves through the King Wen Sequence from one hexagram to the next. The first order of difference will always be an integer between one and six. When the first order of difference within pairs is examined it is always found to be an even number. Thus all instances of first order of difference that are odd occur at transitions from one pair of hexagrams to the next pair. When the complete set of first order of difference integers generated by the King Wen Sequence is examined they are found to fall into a perfect ratio of 3 to 1, three even integers to each odd integer. The ratio of 3/1 is not a formal property of the complete sequence but was a carefully constructed artifact achieved by arranging hexagram transitions between pairs to generate fourteen instances of three and two instances of one. Fives were deliberately excluded. The fourteen threes and two ones constitute sixteen instances of an odd integer occurring out of a possible sixty-four. This is a 3/1 ratio exactly. |
|
Figure 1 shows that when the first order
of difference of the King Wen Sequence is graphed it appears random or
unpredictable. However when an image of the graph is rotated 180 degrees within
the plane and superimposed upon itself it is found to achieve closure at four
adjacent points as shown below:
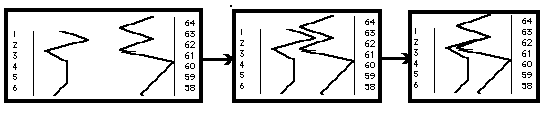 |
Figure 3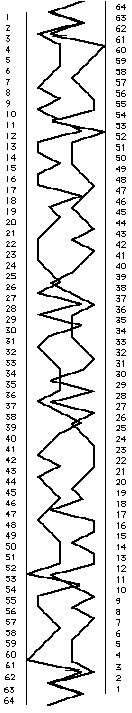 |
While closure might logically be expected anywhere in the
sequence, it in fact occurs at the conventional beginning and end of the
sequence. While an arrangement with closure might have placed any two hexagrams
opposite each other, what we in fact find is that the hexagrams opposite each
other are such that the numbers of their positions in the King Wen Sequence when
summed is always equal to sixty-four. These facts are not coincidences, they are
the artifacts of conscious intent. Over 27,000 hexagram sequences were randomly generated by computer (all sequences having the property possessed by the King Wen sequence that every second hexagram is either the inverse or the complement of its predecessor). Of these 27,000 plus sequences only four were found to have the three properties of a 3/1 ratio of even to odd transitions, no transitions of value five and the type of closure described above. Such sequences were found to be very rare, occurring in a ratio of 1 in 3770. Here is the complete graph of the King Wen first order of differnce with its mirror image fitted against it to achieve closure, as shown in Figure 3. |
| For these reasons I was led to view the King Wen Sequence as a profoundly artificial arrangement of the sixty-four hexagrams. Look carefully at Figure 3 at left. Review in your mind the steps from the King Wen sequence that led to it. Notice that it is a complete set of the sixty-four possible hexagrams, running both sequentially forward and backward. Since it is composed of sixty-four hexagrams of six lines each it is composed of 6 x 64 or 384 lines or yao. One might make an analogy and say Figure 3 is to the King Wen sequence as a cube is to a square; it is composed of the same elements as the King Wen Sequence but it has more dimensions. |
| It is my assumption that the oracle building pre-Han Chinese viewed the forward-and backward-running double sequence of Figure 3 as a single yao or line and that it is therefore open to the same treatment as lines are subject to in the I Ching, namely multiplication by six and sixty-four. |
Figure 4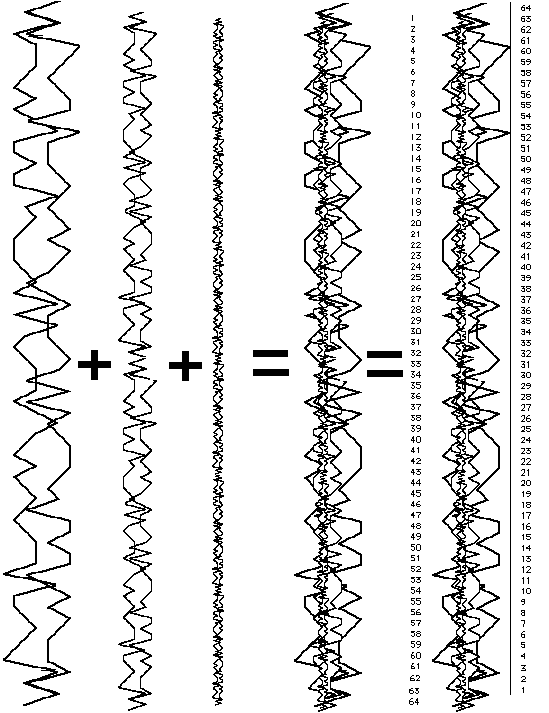
|
| Since a hexagram has six lines I
visualized six double sequences in a linear order. But a hexagram is more than
lines; a hexagram also contains two trigrams. Thus over the six double sequences
I overlaid two double sequences, each three times larger than the six double
sequences. A hexagram also has an identity as a whole; thus over the six and the
two double sequences a single, larger double sequence is projected. The sets of double sequences of each level share a common point of origin and all return to a single end point. The resulting figure, show in Figure 4 on the extreme right, is to the original double sequence as a tesseract is to a cube, for again more dimensions have been added. This figure itself can then be imagined as a single hexagram, but one of a set of sixty-four. |
Figure 5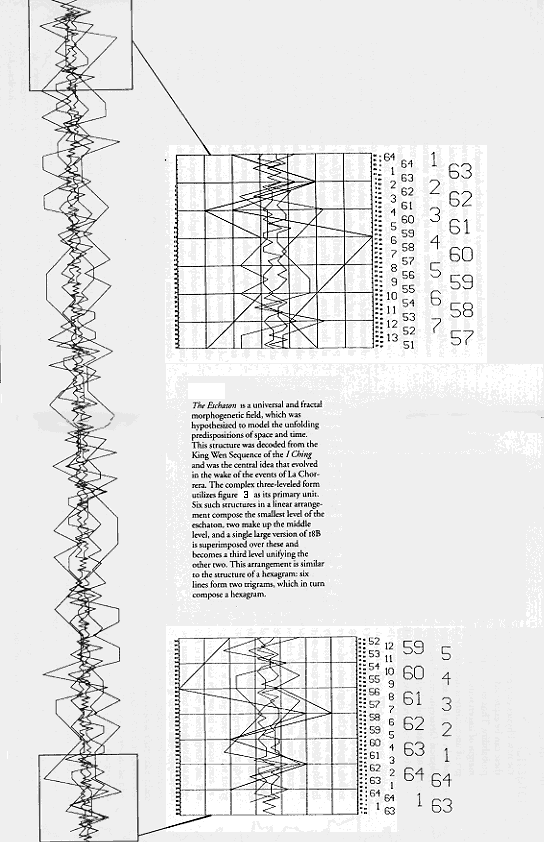
|
| The closure at the beginning and end of this figure suggested that it might be useful to model process. Its 384 subunits imply a calendar. Can it be coincidence that the length of a lunar month, 29.53 days, times 13 is 383.89? I believe that what we have here is a 384 day lunar calendar with resonances to other other naked eye astronomical phenomena known to be of interest to the ancient Chinese (see below). |
Table 1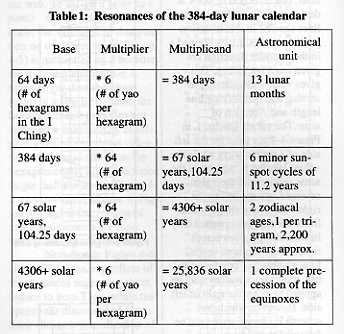
|
|
Using techniques that I developed for the
problem I was able to mathematically collapse the hexagram construct into a
self-similar fractal curve that can be used to map the unfolding of temporal
variables and their resonances on all levels of duration. My attack on the problem began with an examination of the simple wave of Figure 3. Thirteen discrete line types comprise any simple version of the graph. These thirteen lengths are displayed on and off grid as shown below: |
Figure 6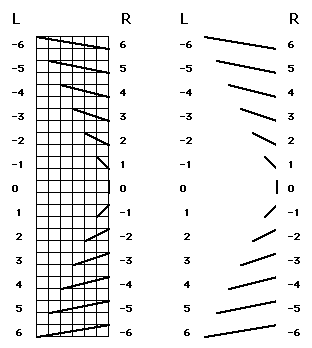
|
Figure 7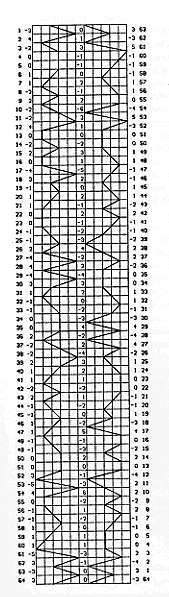 |
As these lengths are always discrete units, we can assign to them values which are ascending integers. The values of Figure 6 allow a quantification of line length. To quantify the degree and direction of skew of individual lines, one direction of skew is designated as positive, giving lines skewed in that direction positive values. Lines skewed in the opposite direction are given negative values. This gives values adequately preserving and quantifying line length and direction of skew. The values labeled L in Figure 6 are used for the left side of a simple wave while the values labeled R, which are the same values with their their sign reverse, are applied to the right side of any simple wave. The sign is important only in combining values across scales but is ignored in the final graphing of combine values, either set of values may be applied to either the right or left side. However, whichever schema is chosen must then be followed throughout. Figure 7 represents the version of these values that we have used for the simple graph. |
|
It is important to note that the
valuations in Figure 7 are valuations of the simple wave on the smallest scale
of a single complex wave. The relative proportions of the three levels in the
complex wave are preserved and quantified by multiplying the valuations of the
linear scale in the appropriate way. To assign a value to a position on the
trigramatic scale, the valuation of that position on the linear scale (Figure 7)
is multiplied by three because the trigramatic scale is three times larger than
the linear scale. In a similar manner, the hexagramatic positions are assigned a
valuation by multiplying their linear-level valuations by six, again because the
hexagramatic scale is six times larger than the linear. Figure 7 uses the value
scheme in Figure 6 and is the version of value assignments we have used in all
our calculations. Note that in Figure 7 all parallel lines, regardless of the distances separating them, reduce to zero. Thus, while the operations discussed so far have allowed quantification of skew direction, proportional ratios of the wave parts, and the degree of departure from the parallel state, they have not provided a quantified account of the fluctuating distances between the two parameters of the wave. The procedure for obtaining these values is similar to, but distinct from, the procedures outlines above.
 Figure 8 shows the seven types of divergence, congruence, and overlap which points in the simple wave may display. The two possible assignments of positive and negative numbers are shown to the right and left sides in Figure 8. We have chosen to use the right-hand schema to preserve the intuition that overlap tends to carry a situation toward the zero state rather than away from it. |
Figure 9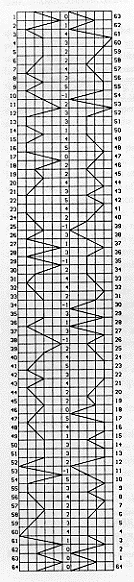 |
Figure 9 shows the values this series of point assignments generates when applied to the simple wave. When the valuations for skew, parallelism, and relative proportion have been combined in the manner detailed above the 384 values (shown in Table 2) result (as read from position 383 to position zero). |
| |
| These same values are to be graphed as a single line graph and are the primary valuation scheme for any complex wave. The process of quantifying a given time in the modular hierarchy of the complex wave will necessarily begin with reference to these values. |
Figure 10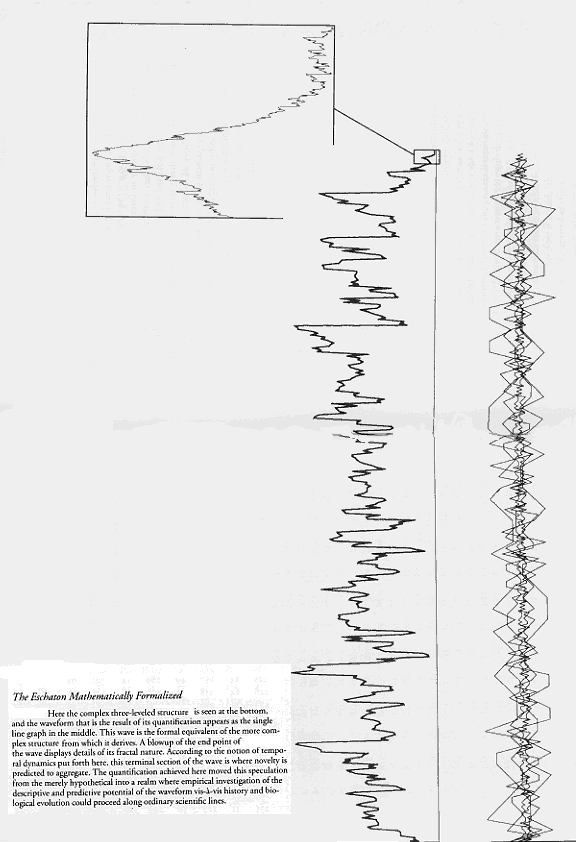 |
Figure 10 shows the complex compound wave and its reduction into an ordinary graph. The 384 values above can be ploted on a graph thus reducing the complex, tri-leveled, bi-directionally flowing complex wave a single line moving in only one direction. Such a graph preserves certain qualities of the complex wave: its divergence from the zero state where lines are parallel, the direction and degree of skew of pairs of lines, the relative proportions of the three levels, and the distances between the fluctuating parameters of the various component waves. However such a graph does not reflect the shift of values that would occur if the single-line complex wave were nested into a particular position in the modular hierarchy of fractal waves each level of which was 64 times larger than its predecessor. In such a case, the 384 values above would serve as a schema of values to be combined through superimposition with the values associated with whichever one of the 64 segments of the next largest level it is to be nested in. These latter values have first been multiplied by by 64, indicating their membership in the next larger level of the hierarchy. Thus 64 variants of the values of Table 2 would be generated, and it is these various waves or frames which we treat as comprising the temporal maps of a given historical span. The values of Table 2 are the basis of the quantified maps of temporal flux which Novelty theory rests on. |
Figure 11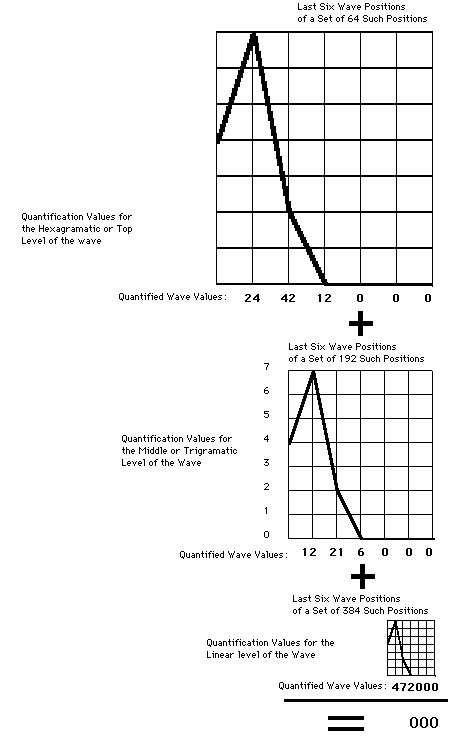 |
The last three of the 384 segments of
the wave on any level possess singularities which quantify as zero, see Figure
11. When the wave on a given level enters those segments of itself which are
zero states, it ceases to contribute boundary constraints to its subsets on
lower levels. The cessation of boundary constraints imposed by higher levels in
the hierarchy causes a "surge" toward the zero state each time that a cycle
enters its terminal phase on any level in the hierarchy. Such quantized
transitions from one modality to another are called "changes of epochs" by
Whitehead. The appearance of life in an inorganic world, of consciousness in an
unconscious world, or of language in a world without language are all examples
of such epochal transitions. Our lives are filled with such transitions, but
they are terminations of relatively short cycles in the quantified hierarchy.
Terminations of cycles or epoches of really long duration cause extreme
accelerations toward the zero state/ This idea is similar to Whitehead's
conception of concrescence and the Vedic conception of world ages which grow
shorter as they tighten around an axis point. The spiral image of the Christian
apocalypse is another example of this intuition that time is a series of
tightening gyres around the quantized emergence of transformation. A perfect self-consistent proof that Table 2 does adequately conserve four qualities--divergence from the zero state where lines are parallel, the direction and the degree of skew of pairs of lines, distances between the parameters of the component waves, and the proportions of these three qualities relative to the other levels--is afforded by comparing any two graph segments assigned to a single time but on different scales relative to an arbitrary termination date that is the same for both. Any two such segments will be found to be similar in form yet preserving their relative proportions. This demonstrable fact confirms the idea that the complex wave derived from Figure 3 is adequately reflected and its useful values retained throughout the series of operations which generate Table 2. What Table 2 and the graphs made from it achieve is a clarity and simplicity of expression lacking in the tri-leveled complex wave. Table 2 and the algorithm that accompanies it is a quantification of operational constructs which makes this modular hierarchy of temporal variables a valid subject for rigorous scientific investigation. |
| Home Page |